\(\text{25}\%\) of \(\text{R}\,\text{124,16}\)
\(\text{25}\% = \frac{\text{1}}{\text{4}}\). \(\frac{\text{1}}{\text{4}} \text{ of } \text{R}\,\text{124,16} = \text{R}\,\text{124,16} \div \text{4} = \text{R}\,\text{31,04}\)
|
Previous
1.6 Ratio, rate and proportion
|
Next
End of chapter activity
|
Let's see how this works in an example.
Use a calculator to answer the following questions:
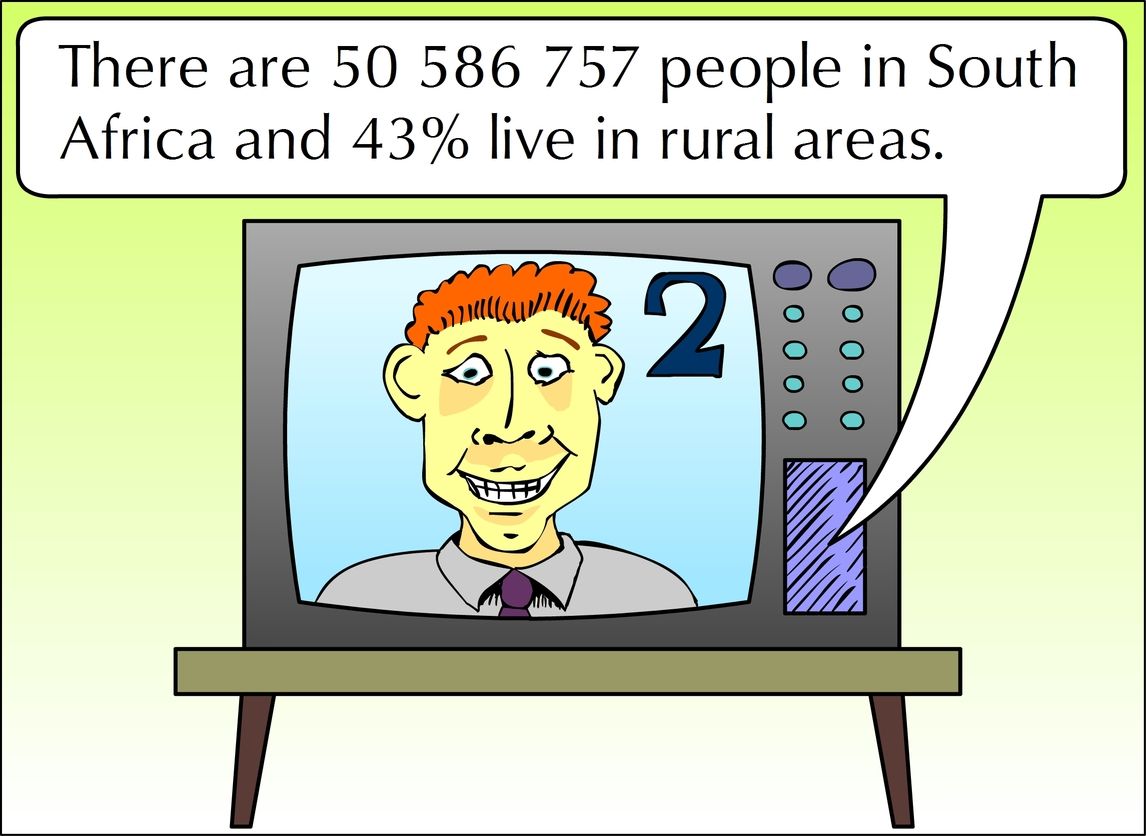 How many people live in rural areas?
How many people live in rural areas?
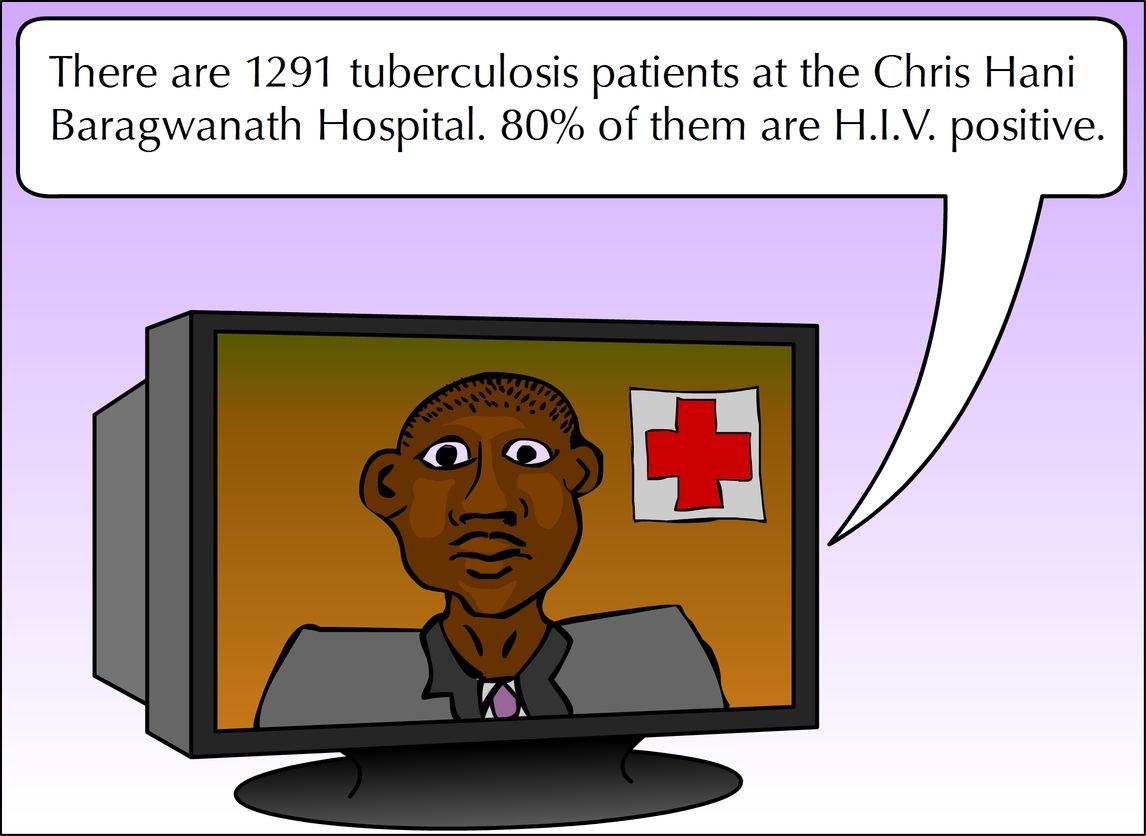 How many T.B. patients are H.I.V. positive?
How many T.B. patients are H.I.V. positive?
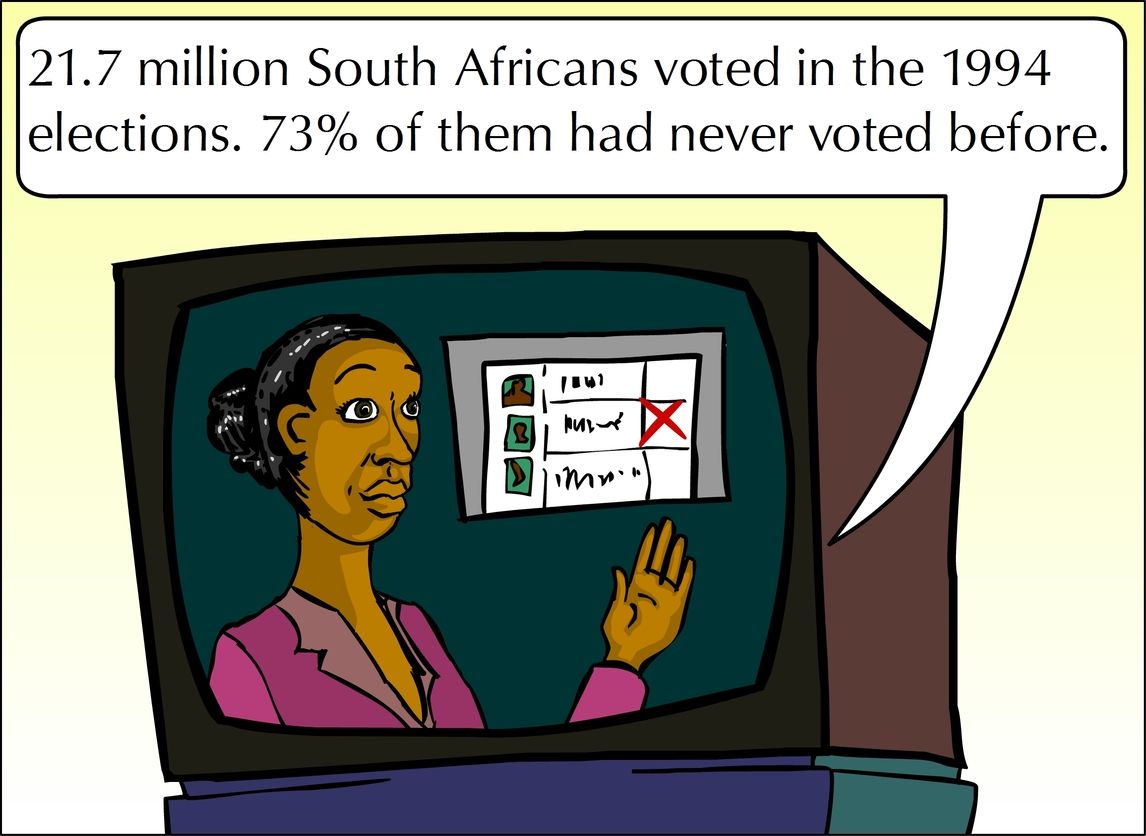 How many people had never voted before the 1994 election?
How many people had never voted before the 1994 election?
Top Teenage T-shirts printed \(\text{120}\) T-shirts. They sold \(\text{72}\) T-shirts immediately. What percentage of the T-shirts were sold?

\(\text{72}\) of the \(\text{120}\) T-shirts were sold
\(\text{72} \div \text{120} \times \text{100} = \text{60}\%\). So \(\text{60}\%\) of the T-shirts were sold.
Calculate the following without a calculator:
\(\text{25}\%\) of \(\text{R}\,\text{124,16}\)
\(\text{25}\% = \frac{\text{1}}{\text{4}}\). \(\frac{\text{1}}{\text{4}} \text{ of } \text{R}\,\text{124,16} = \text{R}\,\text{124,16} \div \text{4} = \text{R}\,\text{31,04}\)
\(\text{50}\%\) of \(\text{30}\) \(\text{mm}\)
\(\text{50}\% = \frac{\text{1}}{\text{2}}\). \(\frac{\text{1}}{\text{2}} \text{ of } \text{30}\text{ mm} = \text{30}\text{ mm} \div \text{2} = \text{15}\text{ mm}\)
Using your calculator and calculate:
\(\text{15}\%\) of \(\text{R}\,\text{3 500}\)
\(\text{R}\,\text{525}\)
\(\text{12}\%\) of \(\text{25}\) litres
\(\text{3}\) litres
\(\text{37,5}\%\) of \(\text{22}\) \(\text{kg}\)
\(\text{8,25}\) \(\text{kg}\)
\(\text{75}\%\) of \(\text{R}\,\text{16,92}\)
\(\text{R}\,\text{12,69}\)
\(\text{18}\%\) of \(\text{105}\) \(\text{m}\)
\(\text{18,9}\) \(\text{m}\)
\(\text{79}\%\) of \(\text{840}\) \(\text{km}\)
\(\text{663,6}\) \(\text{km}\)
Calculate what percentage the first amount is of the second amount (you may use your calculator):
\(\text{25}\%\)
\(\text{8,3}\%\)
\(\text{70}\%\)
\(\text{37,5}\%\)
\(\text{90}\%\)
\(\text{14,3}\%\)
Look at the following extracts from newspaper articles and adverts:

The price of a tub of margarine is \(\text{R}\,\text{6,99}\). If the price rises by \(\text{10}\%\), how much will it cost?
New price is \(\text{R}\,\text{6,99}\) + \(\text{10}\%\) of \(\text{R}\,\text{6,99}\)= \(\text{R}\,\text{6,99}\) + \(\text{70}\) \(\text{c}\) (rounded off) = \(\text{R}\,\text{7,69}\) OR New price is (\(\text{100}\) + \(\text{10}\))\% of \(\text{R}\,\text{6,99}\) = \(\text{110}\%\) of \(\text{R}\,\text{6,99}=\frac{\text{110}}{\text{100}} \times \frac{\text{6,99}}{\text{1}}= \text{R}\,\text{7,69}\) (rounded off)
Top Teenage T-shirts have a \(\text{20}\%\) discount on all T-shirts. If one of their T-shirts originally cost \(\text{R}\,\text{189,90}\), what will you pay for it now?
You only pay \(\text{80}\%\) (\(\text{100}\%\) \(-\) \(\text{20}\%\) discount). Thus: \(\frac{\text{80}}{\text{100}} \times \text{189,901} = \text{R}\,\text{151,92}\) OR \(\text{20}\%\) of \(\text{R}\,\text{189,90} = \frac{\text{20}}{\text{100}} \times \text{189,901}\). The discount is thus \(\text{R}\,\text{37,98}\). You pay \(\text{R}\,\text{189,90} - \text{R}\,\text{37,98} = \text{R}\,\text{151,92}\).
Look at the pictures below. What is the value of each of the following items, in rands?

\(\text{R}\,\text{239,96} - \text{R}\,\text{59,75} = \text{R}\,\text{180,21}\)
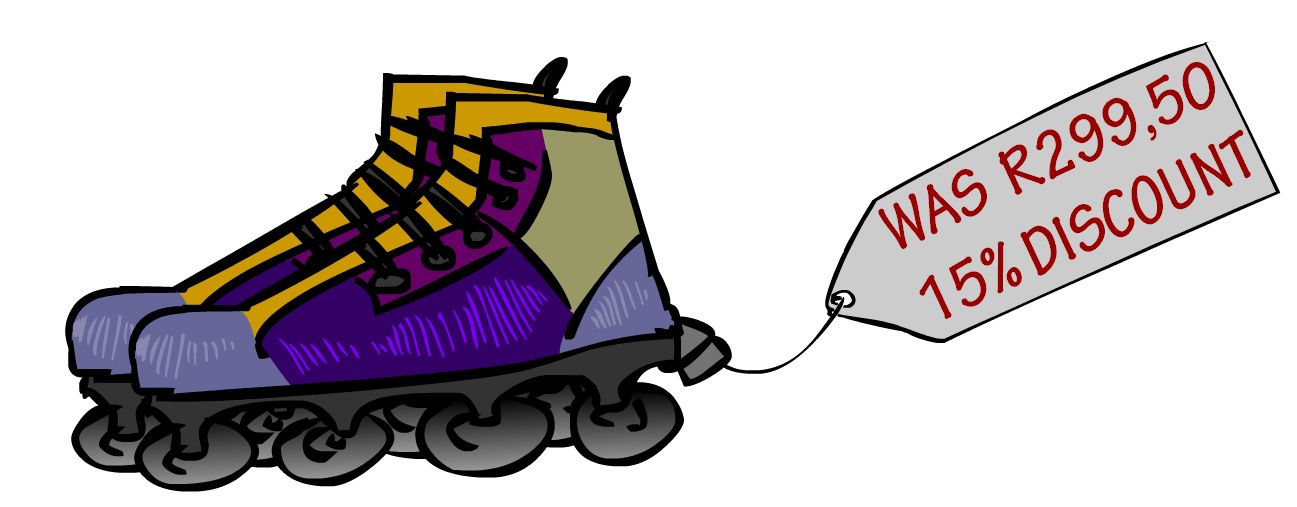
\(\text{R}\,\text{299,50} - \text{R}\,\text{44,925} = \text{R}\,\text{1 254,58}\)

\(\text{R}\,\text{9 875} + \text{R}\,\text{790} = \text{R}\,\text{10 665}\)

\(\text{R}\,\text{15 995} + \text{R}\,\text{799,75}= \text{R}\,\text{16 794,75}\)
Calculate the percentage discount on each of these items:

\(\frac{\text{R}\,\text{1 360}}{\text{R}\,\text{1 523}} = \text{89}\%\). So discount is \(\text{100}\% - \text{89}\% = \text{11}\%\)

\(\frac{\text{R}\,\text{527,40}}{\text{R}\,\text{586}} = \text{90}\%\). So discount is \(\text{100}\% - \text{90}\% = \text{10}\%\)
|
Previous
1.6 Ratio, rate and proportion
|
Table of Contents |
Next
End of chapter activity
|