End of chapter exercises | Euclidean geometry

12.2 Chapter summary

13.1 Area of a polygon

Textbook Exercise 12.2

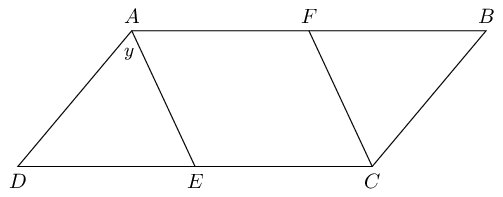
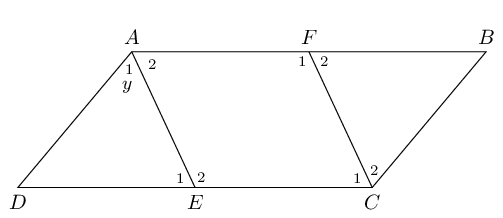
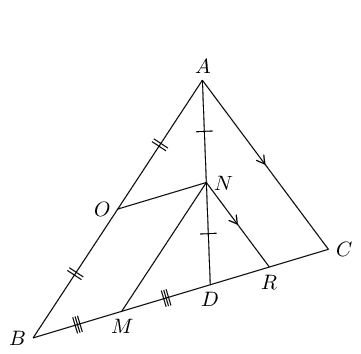
$ABCD$ is a rhombus with $AM = MO$ and $AN = NO$. Prove $ANOM$ is also a rhombus.

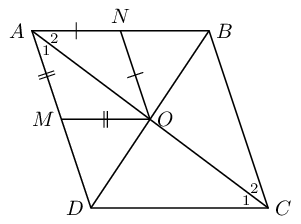
In $\triangle AMO$ and $\triangle ANO$

$\hat{A}_1 = \hat{A}_2$ (given rhombus $ABCD$, diagonal $AC$ bisects $\hat{A}$)

$\therefore \hat{A_1} = A\hat{O}M$ ($\angle$s opp equal sides)

similarly $\hat{A}_2 = A\hat{O}N$

$\therefore \hat{A}_2 = A\hat{O}M$ and $\hat{A}_1 = A\hat{O}N$

but these are alternate interior $\angle$s

$\therefore AN \parallel MO$ and $AM \parallel NO$

$\therefore ANOM$ is a parallelogram

$\therefore AM = NO$ (opp sides of $\parallel$m)

$\therefore AM = MO = ON = NO$

$\therefore ANOM$ is a rhombus (all sides equal and two pairs of sides parallel)

$\triangle AFD \equiv \triangle CHB$

\begin{align*} \hat{A}_1 & = \hat{C}_1 \qquad \text{(alt }\angle \text{s; }AD \parallel BC \text{)}\\ AD & = BC \qquad \text{(opp sides } \parallel \text{m)}\\ AF& = HC \qquad \text{(given)}\\ \therefore \triangle AFD &\equiv \triangle CHB \qquad \text{(SAS)} \end{align*}

$DF \parallel HB$

\begin{align*} \hat{F}_1 &= \hat{H}_1 \qquad (\triangle AFD \equiv \triangle CHB)\\ \therefore \hat{F}_1 +\hat{F}_2 &= 180° \qquad \text{(}\angle\text{s on str line)}\\ \text{and } \hat{H}_1 +\hat{H}_2 &= 180° \qquad \text{(}\angle \text{s on str line)}\\ \therefore \hat{F}_1 &= 180° - \hat{F}_2 \\ \text{and } \hat{H}_1 &= 180° - \hat{H}_2 \\ \therefore 180° - \hat{F}_2 & = 180° - \hat{H}_2 \\ \therefore \hat{F}_2 & = \hat{H}_2 \\ \therefore DF &\parallel HB \qquad \text{(corresp } \angle \text{s equal)} \end{align*}

$DFBH$ is a parallelogram

\begin{align*} FD & = HB \qquad (\triangle AFD \equiv \triangle CHB)\\ \text{and }DF &\parallel HB \qquad \text{ (proved above)}\\ \therefore DFBH & \text{ is a parallelogram (one pair opp sides equal and parallel)} \end{align*}

Write all interior angles in terms of $y$.

First number the angles:

Prove that $AFCE$ is a parallelogram.

\begin{align*} AF &\parallel EC \qquad \text{(opp sides of} \parallel \text{m)}\\ \text {and }\hat{C_1} + \hat{E_2} & = y + (180° - y)\\ \therefore & \text{ the sum of the co-interior angles is } 180°\\ \therefore AE & \parallel FC \\ \therefore AFCE & \text{ is a parallelogram (both pairs opp. sides parallel)} \end{align*}

$XZ$ bisects $\hat{X}$

First label the angles:

$WY = XZ$

\begin{align*} \text{Similarly, }WY &\text{ bisects }\hat{W}\\ \therefore \hat{W_1} &= \hat{W_2}\\ \text{and }\hat{W} &= \hat{X} \text{ (given)}\\ \therefore \hat{W_1} &= \hat{W_2} = \hat{X_1} = \hat{X_2}\\ \text{ and }\hat{W_1} &= \hat{Y_1} ~(\angle \text{s opp equal sides)}\\ \text{In }\triangle WZY&\text{ and }\triangle XYZ\\ WZ&=XY \text{ (given)}\\ ZY &\text{ is a common side}\\ \hat{Z} &= \hat{Y} \text{(third }\angle \text{ in }\triangle \text{)}\\ \therefore \triangle WZY &\equiv \triangle XYZ \text{ (SAS)} \\ \therefore WY & = XZ \end{align*}

Prove that $OBMN$ is a parallelogram.

$AO = OB$ (given)

$AN = ND$ (given)

$\therefore ON \parallel BD$ (Midpt Theorem)

$BM = MD$ (given)

$AN = ND$ (given)

$\therefore MN \parallel AB$ (Midpt Theorem)

$\therefore OBMN$ is a parallelogram (both pairs opp. sides parallel)

Prove that $BC = 2MR$.

$AN = NC$ (given)

$NR \parallel AC$ (given)

$\therefore DR = RC$ (Midpt Theorem)

$\therefore DR = \frac{1}{2} DC$

$MD = \frac{1}{2}BD$ (given)

$\therefore MD + DR = \frac{1}{2} (BD + DC)$

$MR = \frac{1}{2}BC$

$\therefore BC = 2MR$

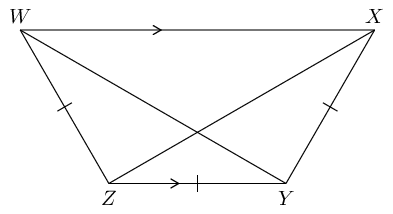
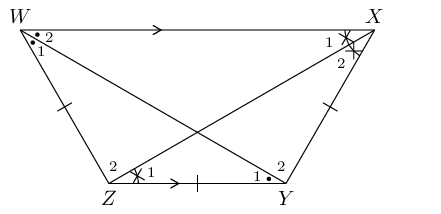
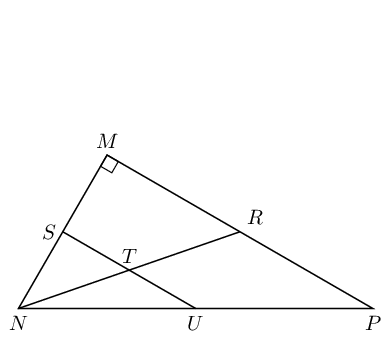
Prove $U$ is the mid-point of $NP$.

\begin{align*} NS&=SM \text{ (given)}\\ NT&=TR \text{ (given)}\\ \therefore ST &\parallel MR \text{ (Midpt Theorem)} \\ \therefore U & \text{ is the mid-point of } NP \text{ (converse of Midpt Theorem)} \end{align*}

If $ST = \text{4}\text{ cm}$ and the area of $\triangle SNT$ is $\text{6}$ $\text{cm$^{2}$}$, calculate the area of $\triangle MNR$.

\begin{align*} N\hat{S}T & = 90° \text{ (corresp }\angle \text{s; } ST \parallel MR)\\ \therefore \text{ area }\triangle SNT &=\frac{1}{2} ST \times SN \\ 6 &= \frac{1}{2}(4)SN\\ \therefore SN &= \text{3}\text{ cm}\\ \therefore MN &= \text{6}\text{ cm} \\ MR &= 2ST = \text{8}\text{ cm}\\ \text{area }\triangle MNR &= \frac{1}{2} MR \times MN\\ &=\frac{1}{2} (8)(6)\\ &= \text{24}\text{ cm$^{2}$} \end{align*}

Prove that the area of $\triangle MNR$ will always be four times the area of $\triangle SNT$, let $ST = x \text{ units}$ and $SN = y \text{ units}$.

\begin{align*} \text{Let }ST \text{ be } & x \text{ units}\\ \therefore MR \text{ will be } & 2x\\ \text{Let }SN \text{ be } & y \text{ units}\\ \therefore MN \text{ will be } & 2y\\ \text{area }\triangle SNT & = \frac{1}{2}xy \\ \text{area }\triangle MNR & = \frac{1}{2} (2x)(2y) \\ & = 2xy\\ \therefore \text{ area } \triangle MNR &= 4\left(\frac{1}{2}xy\right) \\ & = 4(\text{area }\triangle SNT) \end{align*}

Given quadrilateral $QRST$ with sides $QR \parallel TS$ and $QT \parallel RS$. Also given: $\hat{Q} = y$ and $\hat{S} = 63^{\circ}$; $Q\hat{T}R = 38^{\circ}$ and $R\hat{T}S = x$. Complete the proof below to prove that $QRST$ is a parallelogram.

The completed proof looks like this:

\[\begin{array}{|l | l|} \hline \text{Steps} & \text{Reasons} \\ \hline Q\hat{T}R = T\hat{R}S & \text{alt } \angle \text{s; } QT \parallel RS \\ S\hat{T}R = Q\hat{R}T & \text{alt } \angle \text{s; } QR \parallel TS \\ \text{In } \triangle QRT \text{ and } \triangle RST \text{ side } RT = RT & \text{ common side } \\ \therefore \triangle QRT \equiv \triangle STR &\text{congruent (AAS)} \\ \therefore QR = TS \text{ and } QT = RS & \text{congruent triangles} \\ \hat{Q} = \hat{S} & \text{congruent triangles} \\ \therefore QRST \text{ is a parallelogram} &\text{opp sides of quad are } = \\ \hline \end{array}\]

Calculate the value of $y$.

$QRST$ is a parallelogram, $\therefore \hat{Q} = \hat{S}$.

Opposite $\angle$s of parallelogram are equal. $\hat{Q} = \hat{S}$ and $\hat{R} = \hat{T}$.

Therefore, $y = 63^{\circ}$.

Calculate the value of $x$.

$\angle$s in a $\triangle = 180 ^{\circ}$

$\therefore \hat{Q} + Q\hat{R}T + Q\hat{T}R = 180 ^{\circ}$

Now we know that $\hat{Q} = \hat{S} = 63^{\circ}$ and that $R\hat{T}S = 79^{\circ}$.

$\therefore \hat{x} = 180^{\circ} - 63^{\circ} - 79^{\circ} = 79^{\circ}$.

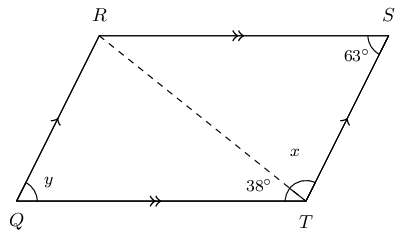
Study the quadrilateral $QRST$ with opposite angles $\hat{Q} = \hat{S} = 117^{\circ}$ and angles $\hat{R} = \hat{T} = 63^{\circ}$ carefully. Fill in the correct reasons or steps to prove that the quadrilateral $QRST$ is a parallelogram.

\[\begin{array}{|l |l|} \hline \text{Steps} & \text{Reasons} \\ \hline R\hat{Q}T = R\hat{S}T & \text{given both } \angle \text{s } = 117^\circ \\ Q\hat{R}S = Q\hat{T}S & \text{given both } \angle \text{s } = 63^\circ \\ \hat{Q} + \hat{R} + \hat{S} + \hat{T} = 360 ^\circ & \text{sum of } \angle \text{s in quad} \\ R\hat{Q}T + Q\hat{T}S = 180 ^\circ & 117^\circ + 63^\circ = 180^\circ \\ \therefore QR \parallel TS & \text{co-int } \angle \text{s; } QR \parallel TS \\ \therefore RS \parallel QT & \text{co-int } \angle \text{s; } RS \parallel QT \\ \therefore QRST \text{ is a parallelogram} & \text{opp. sides parallel} \\ \hline \end{array}\]

Study the quadrilateral $QRST$ with $\hat{Q} = \hat{S} = 149^{\circ}$ and $\hat{R} = \hat{T} = 31^{\circ}$ carefully. Fill in the correct reasons or steps to prove that the quadrilateral $QRST$ is a parallelogram.

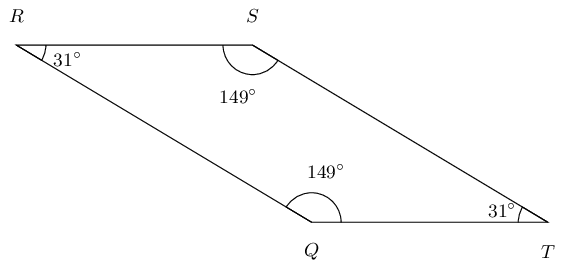
\[\begin{array}{|l |l|} \hline \text{Steps} & \text{Reasons} \\ \hline R\hat{Q}T = R\hat{S}T & \text{given both } \angle \text{s} = 149^{\circ} \\ Q\hat{R}S = Q\hat{T}S & \text{given both } \angle \text{s} = 31^{\circ} \\ \hat{Q} + \hat{R} + \hat{S} + \hat{T} = 360 ^{\circ} & \text{sum of } \angle \text{s in quad} \\ R\hat{Q}T + Q\hat{T}S = 180 ^{\circ} & 149^{\circ} + 31^{\circ} = 180^{\circ} \\ \therefore QR \parallel TS & \text{co-int } \angle \text{s; } QR \parallel TS \\ \therefore RS \parallel QT & \text{co-int } \angle \text{s; } RS \parallel QT \\ \therefore QRST \text{ is a parallelogram} & \text{opp. sides parallel} \\ \hline \end{array}\]

In parallelogram $QTRS$, the bisectors of the angles have been constructed, indicated with the red lines below. You are also given $QT = SR$, $TR = QS$, $QT \parallel SR$, $TR \parallel QS$, $\hat{Q} = \hat{R}$ and $\hat{T} = \hat{S}$.

Prove that the quadrilateral $JKLM$ is a parallelogram.

Note the diagram is drawn to scale.

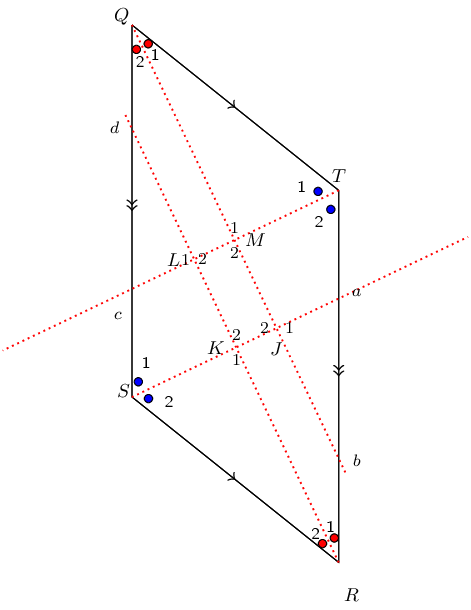
Redraw the diagram and mark all the known information:

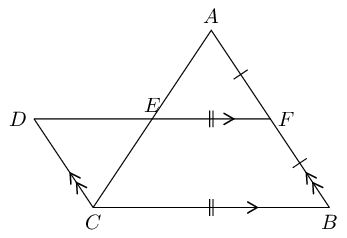
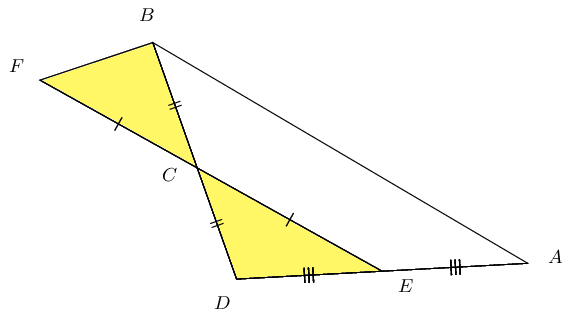
Study the diagram below; it is not necessarily drawn to scale. Two triangles in the figure are congruent: $\triangle CDE \equiv \triangle CBF$. Additionally, $EA = ED$. You need to prove that $ABFE$ is a parallelogram.

Redraw the diagram and mark all known and given information:

Show that $BCDF$ is a parallelogram.

\begin{align*} DF & \parallel CB \text{ (given)} \\ DC & \parallel FB \text{ (given)} \\ \therefore BCDF & \text{ is a parallelogram (both pairs opp. sides } \parallel \text{)} \end{align*}

Show that $ADCF$ is a parallelogram.

\begin{align*} \text{In } \triangle DEC & \text{ and } \triangle FEA \\ C\hat{A}F & = A\hat{C}D \text{ (alt } \angle\text{s; } AB \parallel DC \text{)} \\ A\hat{F}D & = C\hat{D}F \text{ (alt } \angle\text{s; } AB \parallel DC \text{)} \\ DC & = FB \text{ (opp sides parm eq)} \\ \therefore DC & = FA = FB \\ \therefore \triangle DEC & \equiv \triangle FEA \text{ (ASA)} \\ \therefore DE = EF & \text{ and } CE = EA \end{align*}

But $AE$ and $DF$ are diagonals of $ADCF$, $\therefore ADCF$ is a parallelogram (diagonals bisect each other).

Prove that $AE = EC$.

$AE = EC$ (proved above).

$ABCD$ is a parallelogram. $BEFC$ is a parallelogram. $ADEF$ is a straight line. Prove that $AE = DF$.

\begin{align*} BC & = EF \text{ (opp sides of } \parallel \text{m)} \\ BC & = AD \text{ (opp sides of } \parallel \text{m)} \\ \therefore EF & = ED \\ AD + DE & = AE \\ EF + DE & = DF \\ \text{ but } DE & \text{ is common} \\ \therefore AE & = DF \end{align*}

In the figure below $AB = BF$, $AD = DE$. $ABCD$ is a parallelogram. Prove $EF$ is a straight line.

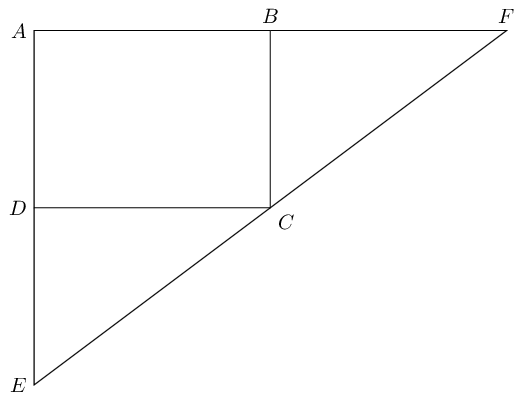
We note that:

\begin{align*} B\hat{A}D & = B\hat{C}D \text{ (opp } \angle\text{s } \parallel \text{m)} \\ C\hat{D}E & = B\hat{C}D \text{ (alt } \angle\text{s; } AE \parallel BC \text{)} \\ F\hat{B}C & = B\hat{C}D \text{ (alt } \angle\text{s; } AF \parallel DC \text{)} \\ \therefore C\hat{D}E & = F\hat{B}C \end{align*}

We also note that:

\begin{align*} AD & = BC \text{ (opp sides parm eq)} \\ AB & = DC \text{ (opp sides parm eq)} \end{align*}

Now we can show that $\triangle DEC$ is congruent to $\triangle BCF$:

\begin{align*} \text{in } \triangle DEC & \text{ and } \triangle BCF\\ C\hat{D}E & = F\hat{B}C \qquad \text{(proven above)} \\ DC = AB & = BF \qquad \text{(given)} \\ DE = AD & = BC \qquad \text{(given)} \\ \therefore \triangle DEC & \equiv \triangle BCF \text{ (SAS)} \end{align*}

Finally we can show that $ECF$ is a straight line:

\begin{align*} \therefore B\hat{F}C & = D\hat{C}E \text{ (}\triangle DEC \equiv \triangle BCF \text{)} \\ B\hat{C}F & = D\hat{E}C \text{ (}\triangle DEC \equiv \triangle BCF \text{)} \\ \text{but } F\hat{B}C + B\hat{F}C + B\hat{C}F & = 180° \text{(sum of }\angle\text{s in } \triangle \text{)} \\ \therefore D\hat{C}E + B\hat{C}F + B\hat{C}D & = 180° \\ \therefore ECF & \text{ is a str line} \end{align*}

12.2 Chapter summary

Table of Contents

13.1 Area of a polygon